Introduction
Most parents of special needs children know that they must understand the law and their rights. Few parents know that they must also understand the facts. The “facts” of their child’s case are contained in the various tests and evaluations that have been administered to the child. Changes in test scores over time provide the means to assess educational benefit or regression.
Important educational decisions, from eligibility to the intensity of educational services provided, should be based on the results of psychological and educational achievement testing. Parents who obtain appropriate special education programs for their children have learned what different tests measure and what the test results mean.
As an attorney who specializes in representing special education children, many parents consult with me after they decide that their child’s special education program is not appropriate. These parents are often right. However, in most cases they do not have the evidence to support their belief, nor do they know how to interpret and use the evidence contained in educational and psychological tests. They need evidence to support their beliefs.
These parents are convinced that a special education program is not providing sufficient help for the child—that under the present special education program, the child is failing to make adequate progress and has fallen further behind. These parents experience a sense of urgency—the child has usually received special education for several years and time is running out.
Critical educational decisions are often based on the subjective beliefs of parents and educators. As a parent, you may believe that your child is not making adequate progress in a special education program. The special education staff may firmly believe that he is doing as well as he can—or that your expectations are too high. Without objective information, both sides take positions that are based on their emotions—and tempered by their hopes and fears. Appropriate educational decision-making must be based on objective information and facts, not subjective emotional reactions and beliefs.
Before you can participate in the development of an appropriate special education program, you must have a thorough understanding of the child’s strengths and weaknesses. This information is contained in tests that are used to measure the child’s abilities and educational achievement.
Tests administered to children fall into several categories: intellectual or cognitive tests; educational achievement tests; projective personality tests, questionnaires and surveys; speech and language tests; and neuropsychological tests.
To successfully advocate for your child, you must also learn about tests and measurements—statistics. Statistics are ways to measure progress or lack of progress, using numbers. After you analyze the scores your child obtains when tested and you know what these numbers mean, you will be able to develop an appropriate educational program for your child—a program that is tailored to the child’s unique needs and from which the child benefits.
As you read this article, you will learn what tests and evaluations measure, how this information is reported, and how to use information from tests to measure and monitor academic progress. You will also learn how to use graphs to visually demonstrate your child’s progress or lack of educational progress in a powerful and compelling manner.
Wrightslaw Note: After you read this article three times, you will be able to interpret and chart your child’s test scores and measure educational progress or lack of progress.
The United States Supreme Court Florence County School District Four v. Shannon Carter (November 9, 1993)
In Florence County School District Four v. Shannon Carter(opens in a new window), 510 U. S.7, 114 S. Ct. 361, (1993), the Supreme Court issued a landmark decision. In Carter, the school system defaulted on their obligation to provide a free appropriate education to Shannon Carter, a child with learning disabilities and an Attention Deficit Disorder. Let’s look at how the courts viewed the facts and the law in the Carter case.
Background
When Shannon was in the seventh grade, her parents talked to the public school staff and expressed their concerns about Shannon’s reading and other academic problems. Shannon was evaluated by a public school psychologist who described her as a “slow learner” who was lazy, unmotivated and needed to be pressured to try harder. Her parents pressured her to work harder. In the ninth grade, despite intense pressure, Shannon failed several subjects.
Shannon’s parents had her evaluated by a child psychologist in the private sector. That evaluator determined that Shannon’s intellectual ability was above average. Educational achievement testing demonstrated that sixteen-year-old Shannon was reading at the fifth grade level (5.4 GE) and doing math at the sixth grade level (6.4 G.E.). Shannon had dyslexia. She was finally found eligible for special education. As she prepared to enter tenth grade, she was also functionally illiterate.
The school district developed an IEP for Shannon’s tenth grade year. This IEP proposed that after a year of special education, Shannon would read at the 5.8 grade equivalent level and perform math at the 6.8 grade equivalent level. In other words, after a full year of special education to remediate her learning disabilities, Shannon was expected to make only four months of progress in reading and math. Progress would be measured by her scores on the Woodcock-Johnson and KeyMath educational achievement tests. She would progress from the 5.4 to 5.8 grade level in reading and from the 6.4 to 6.8 grade levels in math.
Shannon’s parents insisted that their daughter needed a more intensive program so she could learn the necessary reading, writing and math skills. They felt that the proposed program was inadequate, and worried that Shannon would still be functionally illiterate when she graduated from high school three years later. Emory Carter insisted that the school teach his daughter to read, write and do arithmetic at a high school level when she graduated from high school.
Although Emory and Elaine Carter shared their concerns and wishes with the public school officials, the administrators took a “take it or leave it” position. They refused to provide Shannon with a more intensive special education program where she would receive remediation in reading, writing, and arithmetic. The parents requested a special education due process hearing. The Hearing Officer ruled that the public school IEP was appropriate. The parents appealed this decision to a Review Panel. The Review Panel upheld the decision of the Hearing Officer.
At that point, Emory and Elaine Carter withdrew Shannon from the public school and enrolled her in Trident Academy in Mt. Pleasant, South Carolina. Trident Academy is a private school that specializes in educating and remediating children with learning disabilities, including dyslexia. When Shannon graduated from Trident Academy three years later, her reading and math scores were on a high school level.
Shannon’s parents then appealed the Review decision to the U. S. District Court. They asked Judge Houck to award them reimbursement for Shannon’s private school education at Trident.
After hearing testimony and reviewing the transcripts and documents from the Due Process and Review Hearings, Judge Houck found that the school district’s IEP was “wholly inadequate” to meet Shannon’s needs. He ruled that Shannon had received an appropriate education at Trident and ordered Florence County to reimburse Shannon’s parents for the costs of her education. (Read Judge Houck’s decision(opens in a new window))
On what basis did Judge Houck decide that the IEP proposed by Florence County was inappropriate? What evidence caused him to decide that Shannon received an appropriate education at Trident Academy?
Evidence & law
The decisions in Shannon’s case, and in most special education cases, are based on the evidence provided by tests and evaluations of the child. When Judge Houck wrote that Florence County’s IEP was “wholly inadequate” to meet Shannon’s needs, he was relying on test results. Judge Houck knew the importance of accurately interpreting test scores. He charted Shannon’s test scores and included this information in his decision(opens in a new window). (See also Hall v. Vance, 555 EHLR 437, (E.D. NC 1983), affirmed at 774 F. 2d 629, 557 EHLR 155, (4th Cir. 1985)) in which U. S. District Court Judge Dupree charted out James Hall’s test scores to support his decision that Vance County, North Carolina did not provide James with an appropriate education.)
Florence County appealed Judge Houck’s decision to the U. S. Circuit Court of Appeals for the Fourth Circuit. Appeals from the U. S. District Courts in Maryland, Virginia, West Virginia, North Carolina and South Carolina are heard in the U. S. Court of Appeals for the Fourth Circuit by a three judge panel. Appeals from U. S. Circuit Courts of Appeals are filed in the U. S. Supreme Court. Occasionally a U. S. Court of Appeals will convene all Judges appointed to the Circuit to hear a case. This is called an en banc review.
A three judge panel of the Fourth Circuit affirmed Judge Houck’s decision(opens in a new window) about the inadequacy of Florence County’s proposed IEP. Florence County then appealed to the United States Supreme Court.
On October 6, 1993, Pete Wright argued Shannon’s case before the Court. Thirty-four days later, on November 9, 1993, the Supreme Court issued a unanimous favorable decision(opens in a new window) on Shannon’s behalf. In the Carter decision(opens in a new window), written by Justice Sandra Day O’Connor, the Court upheld the lower court decisions, ruled against Florence County School District Four, and ordered them to reimburse Shannon’s parents for the costs of her tuition, room and board, and attorney’s fees.
Legal requirements: Measure progress objectively
The Individuals with Disabilities Education Act(opens in a new window) (IDEA) requires IEPs to include measurable annual goals and a description of how the child’s progress toward meeting the annual goals will be measured.
(A) Individualized Education Program.
(i) In General. The term ‘individualized education program’ or IEP means a written statement for each child with a disability that is developed, reviewed and revised…and that includes:
(II) a statement of measurable annual goals, including academic and functional goals, designed to
(aa) meet the child’s needs that result from the child’s disability to enable the child to be involved in and make progress in the general education curriculum; and
(bb) meet each of the child’s other educational needs that result from the child’s disability;(III) a description of how the child’s progress toward meeting the annual goals described in subclause (II) will be measured and when periodic reports on the progress the child is making toward the annual goals … will be provided … (See 20 U.S.C. 1414(d)(1)(A)(i)(II and III; page 99 in Wrightslaw: Special Education Law, 2nd Edition, page 99. See also the “Definition of individualized education program” in the federal special education regulations, Volume 34 of the Code of Federal Regulations, 34 CFR 300.320(a)(2)(i) and (a)(3)(i); page 245 in Wrightslaw: Special Education Law, 2nd Edition)
Wrightslaw Note: Shannon’s case was decided under an earlier authorization of the Individuals with Disabilities Education Act. Shannon’s IEP stated that she “will be able to improve total reading level from the 5.4 grade level to the 5.8 grade level as measured by the Woodcock Reading Mastery Test … (and that she) will improve math skills from the 6.4 grade equivalent to the 6.8 grade equivalent as measured by the Key Math Diagnostic Test.” This IEP complied with the regulation in existence at that time, (34 C.F.R. § 300.346, since modified), by including “appropriate objective criteria.” The IEP required that the Woodcock-Johnson and KeyMath tests be readministered to measure Shannon’s progress.
The U. S. District Court and the Fourth Circuit found that the proposed gain of four months after a full year of special education was “wholly inadequate.”
In an effort to avoid Florence County’s fate, many school districts around the country develop IEPs that include no objective measures of the child’s progress. Instead of developing goals where the child’s progress is measured using objective tests, as Florence County did, many schools now devise IEPs that rely subjective teacher observations of the child’s progress. Let’s see how this works.
Johnny is a child who has a learning disability that is affects his ability to read. In reading, Johnny is below grade level. Instead of developing an IEP that will measure his progress in reading on specific objective tests, the special education staff may write a goal like this: “Johnny will make measurable progress in reading, as measured by teacher observation and teacher made tests at 80% accuracy.”
In that case, “objective measurement of progress” becomes the teacher’s subjective observation about whether her student made progress in reading, writing, or arithmetic. The criteria for determining progress becomes 80% of a subjective belief or opinion. When parents object and request a more intensive program that includes clear objective scores, they are often rebuffed or criticized.
In many areas, school board counsel and state departments of education advised schools to stop using objective measurements of progress (tests) to measure progress for special education children.
If you believe that the special education your child is receiving is inadequate, you must have evidence to support your position. You will find this evidence in the public school and private sector testing that has been or will be completed on your child.
To master the material in this article, you should expect to read the article at least three times. When you take this step, you will know what tests and evaluations measure and how test results are reported. You will know how to convert scores on different tests into numbers that are easily understood. And, you will know how to measure a child’s educational progress or lack of progress, i.e. regression.
Michael
Three years ago, your eight-year-old son Mike began to have serious difficulties in school. By the time he entered third grade, you were deeply concerned about his difficulties in learning to read. His handwriting was nearly illegible. Homework was a nightmare. You consulted with Mike’s teacher about these problems several times. Eventually, the teacher sent Mike’s “case” to a special education committee. You attended a meeting of this committee. The committee recommended that Mike be evaluated through the school’s special education department. Relieved that something would be done to help you child, you consented to these tests.
According to the evaluations, your son has a learning disability. In Mike’s case, he has visual-perceptual problems and visual-motor problems that negatively affect his ability to read and write. Based on the results of the evaluations, your son was found eligible for special education services at his neighborhood school.
After Mike was found eligible for special education, you attended a meeting to develop his Individualized Education Program (IEP). This IEP provided for Mike to receive one period of special education in an “LD Resource” class every day. It was your understanding that Mike would receive individualized help in reading and writing from a teacher who was specially trained to remediate his learning disability problems.
Three years have passed. Mike hasn’t made much progress, despite the special education help. He still has difficulty reading aloud. His spelling is poor, and his handwriting is unreadable. He is behind most of the children in his class. His attitude has changed. He is angry and depressed and says he “hates school.”
When you discussed your concerns about Mike’s lack of progress with his special education teacher, she reassured you that he was “making progress” and told you to be patient. From your perspective, patience is not the issue. You are worried that your son will never master the basic academic skills. You are worried about his future.
At a recent IEP meeting, you reiterated your concerns about Mike’s lack of progress and expressed your belief that he needs more help than he is getting in the school’s Resource program. The IEP team disagreed with you. One person said that Mike was getting all the help he needs and that he was really doing quite well. Another member said that your expectations were too high—and if you don’t accept Mike’s “limitations,” you will damage him emotionally.
What should you do?
You know that time in the LD resource class with several other children is not providing Mike with the individualized help he needs. The school is not teaching your son how to read, write and do arithmetic. Instead, his IEP team wants to lower the bar. They suggest more “accommodations” and “modifications.” They propose to reduce his workload, give him untimed tests, and provide him with “talking books” and a calculator. They do not propose to give him the individualized help he needs so he can learn to read, write, and do arithmetic.
You believe that Mike’s new “emotional problems” are caused by shame and embarrassment because he is not successful in school. How can you, a parent, prove this to the staff at Mike’s school so that they will develop an appropriate educational program for him? How will you know when he is getting the help he needs
The process of educational decision-making
Legal requirements: Measure progress objectively
Many parents assume that interpreting their child’s test data is beyond their competence, that this is the responsibility of the school personnel. If parents do not accept the responsibility of learning this information, they leave interpreting the test data to the school psychologist. The school psychologist often has very little information about your child, aside from scores on tests administered years ago.
The basic principles of tests and measurements are not difficult to learn. As you read this article, you will see that you are already familiar with many of the concepts discussed. Statistics and statistical terms are used in many other areas of life, from business and sports to medicine. Newspaper and magazine articles use statistics to inform readers about change or lack of change. You read articles about changes in the population, the climate, the economy. Even public opinion polls include statistical information to inform you or persuade you of a point.
As a parent, you need to expend time and effort to develop a basic understanding of statistics. To accomplish this goal, you should reread parts of this article several times. Underline, make margin notes, and use a highlighter. Be patient and put in the time. The time you spend now will help to change your child’s life forever.
As you study this material, you are likely to see some terms and concepts that are confusing at first –terms like standard deviation, standard scores, and grade and age equivalents. Other concepts will be familiar—averages, percentages.
When you master this information, you will understand the educational and psychological tests administered to your child. You will be able to use this information to make wise decisions about your child’s education. You’ll find that your newfound knowledge and expertise exceeds that of many of the special education staff and IEP team members.
When you attend your next IEP or Eligibility meeting, you will be glad you did your homework!
Katie
Katie is fourteen years old and in the ninth grade. She “hates school” and is failing several subjects. As a young child, Katie was bright, happy, and curious. When she entered third grade, her attitude began to change. Now, she locks herself in her room, lies on her bed, and listens to music for hours. She is sullen and angry and says she can’t wait to quit school.
In desperation, Katie’s parents took her to a child psychologist for testing. At a meeting to interpret the test results to Katie and her parents, the psychologist explained that Katie scored two “standard deviations” above the mean on the Similarities subtest of the Wechsler Intelligence Test for Children, Fourth Edition (WISC-IV) and two and a half “standard deviations” below the mean on the spontaneous writing sample of the Test of Written Language, Third Edition (TOWL-3).
Wrightslaw Note: Test publishers update and revise their tests fairly often. This article does not focus on any test’s strengths or weaknesses, since weaknesses are often corrected in the next edition of that test.
The Wechsler Intelligence Scale for children was originally known as the WISC. Later, it was revised and called the WISC-R. Several years later, the next version was published as the WISC-III. The current version is the Fourth Edition or WISC-IV. The first Test of Written Language (TOWL) was replaced by the TOWL-2, then revised and replaced by the TOWL-3.
The Woodcock Johnson battery of tests was known as the Woodcock Johnson Psycho-Educational Battery. The WJPEB included educational achievement testing and cognitive ability testing. The current version is called the Woodcock-Johnson III Tests of Achievement (WJ-III) measures academic achievement. The Woodcock-Johnson III Tests of Cognitive Abilities (WJ III) measures general intellectual ability and specific cognitive abilities. Dr. Woodcock also produced the Woodcock Reading Mastery Test, now called the Woodcock-Johnson III Diagnostic Reading Battery (WJ III-DRB).
Tip: For information about thousands of tests, go to Testlink(opens in a new window) from the Educational Testing Service (ETS).
Parents are often surprised to learn that tests do not necessarily measure what they purport to measure. As you will see, a child’s score on a push-up test can be represented as an overall fitness score, a measure of arm strength, an upper body measurement score, a measure of perseveration and persistence, or a measure of a child’s motivation. A score may measure only one variable or it may accurately reflect all of the above.
Let’s look at tests that measure reading ability. One test that purports to measure a child’s reading ability actually measures the child’s ability to correctly read aloud and pronounce isolated words out of context, i.e., a word recognition test. This test includes a list of words, i.e., cat, tree, dog, house, person, etc. It does not measure reading and may be adversely affected by a child’s speech or word finding problems.
Another reading test has the child read a passage of text, then answer a series of multiple choice questions about the passage. In this case, the score may be a measure of the child’s ability to eliminate certain answers in the multiple choice format, i.e., reasoning, not reading. Some very bright children may need to recognize and interpret only a few words to discern the total context. Others have excellent word recognition abilities but cannot link or interpret the words in a body of text or passage.
Another reading test has the child read a passage of text aloud (measuring oral reading), then answer questions. The accuracy of the words read aloud and the child’s understanding of the passage makes up the reading score.
Tip: You need to know what tests were used and what the tests measure. To learn more about reading tests, read Reading Tests: What They Measure, and Don’t Measure by Dr. Melissa Farrall.
When we first discussed Katie, we saw that she scored two “standard deviations” above the mean on the Similarities subtest of the Wechsler Intelligence Test for Children, Fourth Edition (WISC-IV) and two and a half “standard deviations” below the mean on the spontaneous writing sample of the Test of Written Language, Third Edition (TOWL-3).
Do these test scores explain the academic problems Katie is having? Do they have anything to do with her moodiness and her intense dislike of school? (Answers: Yes and Yes.)
When we return to Katie’s case later in this article, you will understand the significance of her test scores. You will also understand why Katie’s self esteem has plummeted.
After you learn the material in this article, you will be able to interpret your child’s test scores. You will be able to read the preceding paragraph and understand the significance of Katie’s scores. You will have acquired skills that will allow you to answer questions like these:
- How is your child functioning, compared with other children the same age?
- How is your child functioning, compared with others in the same grade?
- How much educational progress has your child made (what has been learned) since the last test battery?
- If your child is receiving special education, has the child progressed or regressed in the special education placement?
- If your child has shown an increase in age and grade equivalent test scores, has the child actually fallen further behind the peer group?
You will also learn how to include objective measurements in your child’s IEP so the child’s educational progress can be charted often.
Measuring change: Rulers, yardsticks and other tools
To clarify these points, let’s change the facts. You can measure your child’s physical growth with a measuring tape and a bathroom scale. You can measure growth by charting how much height increases, as measured in inches, and how much weight increases, as measured by pounds, over a period of months or years. Using these tools, you can document his physical growth. You don’t need to be a doctor to understand that increases in these measurements prove that your child is growing.
Assume that your child’s height was five feet, three inches last year. This year, the child is five feet, six inches tall. You can report this information in several ways. You can say that last year, your child was sixty-three inches tall and is now sixty-six inches tall. Or, you can say that your child was 5.25 feet tall and is now five and a half feet tall. You can even say that a year ago, your child was 160 centimeters tall and is now 168 centimeters tall. Or, that your child was 1.75 yards tall and is now 1.83 yards tall!
If you or your child’s pediatrician have measured your child’s height and weight at regular intervals, you can create a chart or graph that documents changes in height or weight over time. Your child’s pediatrician has growth charts you can use to compare your child’s growth with the growth of the “average” child.
Academic or educational growth can be measured and charted too. The yardsticks used for measurement are different, but the principles are the same. Measuring educational growth or progress is not much different from measuring physical growth. Instead of a tape measure and scales, you need the psychological and educational achievement test results. Where will you find the information you need? How can you measure change?
Most school districts test their students on standardized educational achievement tests at regular intervals. The results of these tests provide information about how well the school district is accomplishing the mission of educating children. The information contained in group standardized tests can provide you with some basic information.
Standardized educational achievement tests are general measures. The information they provide is similar to that provided by medical screening tests. Medical screening tests can suggest that a problem exists. In most cases, additional testing is necessary before the problem can be accurately identified and a treatment plan developed. Children’s learning problems are identified in a similar manner. In most schools, individual ability and achievement tests that identify academic or functional problems are administered by school psychologists and educational diagnosticians.
What do evaluations tell you?
As you continue on your advocacy journey, you need to understand the exact nature of your child’s disabling condition(s). How does the disability affect her? In what areas? How serious is it? What are her strengths and weaknesses? Does she need special education? What educational issues need to be addressed? How will you know if she is making progress? How much progress is sufficient?
The answers to these questions will be found in the evaluations and tests that are administered to children and adolescents.
Many parents believe they cannot understand these tests. Usually, their reasoning goes like this:
Gosh. I’m just a parent. I didn’t even finish college. I don’t have any training in education or special education so I can’t understand that stuff!
or
The people who did that testing on my kid went to school for years to learn how to do that. Who am I to think I can understand it? I’m not a psychologist!
If you believe that you “can’t” understand your child’s testing, it’s time to change your beliefs. You may be reading this article because your son or daughter is performing poorly in school—or has been identified with learning problems—and now believes that he or she “can’t” read or write or do arithmetic. Your child must overcome these false beliefs about learning new or difficult material. And, so must you.
Statistics: General principles
Statistics are simply ways to measure things and to describe relationships between things, using numbers. Part of the confusion that many people experience when they begin to learn statistics is because of the terms and concepts are unfamiliar. As we learned in the earlier discussion about measuring physical growth, there are several ways to report the same information (inches, feet, yards, centimeters, etc.) This can be confusing.
First, let’s look at a familiar situation that many of us deal with regularly—how to measure our car’s gas mileage. Remember: When we use statistics, we can use several terms to describe the same concept. If you want to describe your car’s gas mileage, you can make any of the following statements:
- My gas tank is half full.
- My gas tank is half empty.
- I am at the fifty percent mark.
- My odometer shows that I have another 150 miles before the next fill-up.
- My odometer shows that I have traveled 150 miles since I last filled the tank.
All of these statements accurately describe your car’s consumption of gas.
When you have this information, you can make decisions. When will you need to buy more gas? You know that your car has a 15 gallon gas tank. According to the gas gauge, your tank is slightly below the halfway mark. You’ve been driving in the city. You’ll be driving on the highway for the rest of your trip. You have used a precise amount of gas and have a precise amount of gas left in your tank. You can describe and define this information in several ways—gallons used, gallons remaining, miles driven, miles to go, percentage full, and so forth. Using this information, you can do some simple math calculations to learn that your car averages between 17 to 23 miles to a gallon of gas, depending on driving conditions.
Using this information or data, you can also measure change. If you compare your car’s present or current mileage to the mileage you obtained last month, before you had your car tuned up, you can measure miles per gallon before and after the tune-up. In this way, you can measure the impact of the tune-up on your car’s gas consumption. You can also compare your car’s mileage performance to that of other vehicles.
Let’s look at another common way in which we use tests and measurements. When you last visited your doctor, you mentioned that you were feeling tired and sluggish. Your doctor asked several questions, then recommended that you have some lab work. After reviewing the test results, the doctor explained that your blood glucose level was moderately elevated.
To lower your blood glucose level, the doctor recommended a plan of treatment that included a special diet and a daily program of moderate exercise. After a month, you return for a follow-up visit. More lab work is completed. If your glucose level has returned to normal, it is unlikely that you will require additional treatment. But, if your glucose level remains high despite the diet and exercise program, you may need more intensive treatment. By measuring change after an intervention with “data based documentation of repeated assessments … at reasonable intervals, reflecting formal assessment of progress” (34 C.F.R. 300.309(b)(2), you and your doctor can make rational decisions about your medical treatment.
Remember: The principles that allow you to compute your car’s gas mileage and make medical decisions will also allow you to measure and monitor educational progress. When you measure educational progress (as when you measure your gas mileage and blood levels), the test scores can be reported and compared in several different ways.
Because educational test scores are often reported in different formats and compared in different ways, it is essential for parents and advocates to understand all of the scoring methods used in measuring and evaluating educational progress, including:
- age equivalent scores (AE)
- grade equivalent scores (GE)
- standard scores (SS) and standard deviations (SD)
- and percentile ranks (PR).
Knowledge about statistics will enable you to assess your child’s progress or lack of progress in a particular educational program. Lack of progress is usually referred to as regression. Unfortunately, regression is a common educational problem that we will discuss in more detail later. You must learn how to recognize regression and reverse the downward spiral before your child is further damaged.
Applied statistics
Let’s look at the performance of a group of children. You need to understand how an individual child scores when compared with other children who are his age or in his grade—and what this means.
First, we’ll examine a single component of physical fitness in a group of elementary school students. Our group or sample consists of 100 fifth grade students. These children are enrolled in a physical fitness class to prepare them to take the President’s Physical Fitness Challenge. We will assume that the average chronological age (CA) of these children is exactly ten years, zero months. (CA=10-0) The children are tested in September, at the beginning of the school year.
To qualify as “physically fit,” each child must meet several goals. Push-ups are one measure of upper body strength. Each child must complete as many push-ups as possible in a period of time. Each child’s raw score is the number of push-ups completed. The term raw score is simply another way of describing the number of items correctly answered or performed.
After all of the fifth grade students complete the push-up test, their scores are listed. The results are as follows:
- Half of the children completed ten push-ups or more.
- Half of the children completed ten push-ups or less.
- The average child completed 10 push-ups.
- The average or mean number of push-ups completed by this class of 100 fifth grade students is 10.
- Half of the children scored above the mean score of 10.
- Half of the children scored below the mean or average score of 10.
- 50 percent of the children scored 10 or above.
- 50 percent of the children scored 10 or below.
As we continue to analyze the children’s scores, we see patterns:
- One-third of the children scored between 7 and 10 push-ups.
- One-third of the class completed between 10 and 13 push-ups.
- Two-thirds of the children scored between 7 to 13 push-ups.
- Half of the children (50 percent) completed between 8 and 12 push-ups.
- The lowest scoring child completed 1 push-up.
- The highest scoring child completed 19 push-ups.
Again, two-thirds of the children in this fifth grade class were able to complete between 7 and 13 push-ups. The remaining third of the children did fewer than 7 or more than 13 push-ups. Nearly all of the children—98 out of 100—were able to complete between 4 and 16 push-ups. Click here to view the bell curve chart.
The test results provide us with a sample of data. As we analyze the data in our sample, we can compare the performance of any individual child with that of the entire group. As we make these comparisons, the data will enable us to recognize any child’s strengths and weaknesses when compared with the peer group of similar youngsters.
If we conduct an identical push-up test with children in other grades, we can compare our original group of 100 fifth grade children with other groups of youngsters—children who are older, younger, in different grades, in different schools. If we gather enough information or data from other sources, we can compare our original group of fifth graders—or an individual child within our group—to a national population of children who are being tested for their upper body strength as measured by their ability to do push-ups.
Using the bell curve to measure progress
In nature, traits and characteristics distribute themselves along theoretical curves. For our purposes, the most important curve is called the normal frequency distribution or bell curve. Because the percentages along the bell curve are well-known and thoroughly researched, they become our frame of reference.
By using the bell curve, we can develop a diagram or graph of the children’s push-up scores. This map—on the bell curve—provides us with additional information. We can see what percentage of children were able to complete specific numbers of push-ups. When we use the bell curve, we can visually demonstrate where any particular child scores, when compared with other children who are the same age or in the same grade. Likewise, with educational test scores, we can visually demonstrate scores and change over time.
If we compare the push-up scores obtained by children who attend different schools, we can determine whether the physical fitness of children, as measured by their ability to do push-ups, varies in different schools, neighborhoods, states, or countries.
We can also measure progress over time—with push-ups and with improvement in reading skills. Let’s look at our class of fifth graders again. We want to gather information as to whether the physical fitness class is effective—whether the children’s fitness levels improve. How can we answer this question?
To measure the effectiveness of the fitness class, we will measure the children’s number of push-ups before they take the class and compare this score with their score after they take the class. If the class is effective, we should see individual improvement and group improvement. Some children will have minimal improvement—these children will fall further behind the peer group. Other children who performed below their peers may show significant improvement. Some children will improve so much that they now perform as well or better than the “average” youngster.
We will measure the children’s progress on one or more occasions as they progress through the class. If the fitness class is “working,” that is, if the children’s’ fitness levels are improving, their ability to perform fitness skills should improve measurably over time. In our example, physical fitness improvement is being measured with “technically sound instruments” that “are valid and reliable” (34 C.F.R. §300.404(b)(c)) and use “Data-based documentation of repeated assessments of achievement at reasonable intervals, reflecting formal assessment of student progress …” (34 C.F.R. §300.309(b)(2))
Because of its value and usefulness in measuring educational progress, we will return to the subject of the bell curve repeatedly throughout this article.
The bell curve: basic concepts
On all bell curves, the bottom or horizontal line is called the X axis. In our sample of fifth graders, the X axis represents “number of push-ups.” And, on all bell curves, the up- and- down vertical line is called the Y axis. In our sample, the Y axis represents the number of children who earned a specific score (number of push-ups completed).
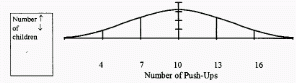
As you can see in the diagram above, the highest point of the bell curve on the X axis is equal to a score of 10 push-ups. You recall that more children completed 10 push-ups than any other number. Thus, the highest point on this bell curve represents a score of 10. The next most frequently obtained scores were 9 and 11, followed by 8 and 12. This pattern continues out toward the far ends of the bell curve. In our example, the ends occurred at 1 and 19 push-ups.
Using the bell curve, we can now chart each child’s score and compare it to the score achieved by all 100 students in the class. Look at the bell curve above, and find 10 push-ups. We know that Amy completed 10 push-ups so her raw score was 10. Ten push-ups placed her squarely in the middle of the class. Half of the youngsters in Amy’s class earned a score of 10 or more; half of the children scored 10 or less. If you look at the bell curve diagram (below), you see that Amy’s score of 10 placed her at the 50% level. The individual’s percent level is referred to as their percentile rank (PR). Amy’s percentile rank is 50 (PR=50).

Erik completed thirteen push-ups. Looking at the bell curve above, you see that his score of 13 placed him at the 84th percent level. Erik’s percentile rank is 84 (PR=84). Erik’s ability to do push-ups placed him at the 84th position out of the 100 fifth grade children tested on our measure of upper body strength.
Sam completed seven push-ups. His raw score of 7 placed him at the (bottom) 16 percent. Sam’s percentile rank was 16 (PR=16). Out of our sample of 100 fifth grade children, 84 children earned a higher score than Sam.
Larry completed 6 push-ups. We can convert his raw score of 6 to a percentile rank of 9 (PR=9). 91 children scored higher and 8 children scored lower than Larry in upper body strength as measured by the ability to do push-ups.
Oscar completed 2 push-ups. His raw score of 2 placed him in the bottom 1 percent of fifth graders tested (PR=1).
Nancy’s raw score of 17 placed her at the upper 99 percent. We say that Nancy scored at the 99th percentile rank (PR=99).
You can see the relationship between the number of push-ups completed and the child’s percentile rank (PR) reproduced in the table below:
| Push-ups | Percentile Rank | Push-ups | Percentile Rank |
|---|---|---|---|
| 19 | 99 | 9 | 37 |
| 18 | 99 | 8 | 25 |
| 17 | 99 | 7 | 16 |
| 16 | 98 | 6 | 9 |
| 15 | 95 | 5 | 5 |
| 14 | 91 | 4 | 2 |
| 13 | 84 | 3 | 1 |
| 12 | 75 | 2 | 1 |
| 11 | 63 | 1 | 1 |
| 10 | 50 |
The bell curve is a powerful tool. When you use the bell curve, you can objectively compare any child’s percentile rank to that of a group of children. You can also compare a single child’s progress or regression when compared to the group.
Using the bell curve, you can compare a single child’s score to the scores obtained by other children who are older or younger or in different grades.
Let’s see how this works. Again, we will measure the children’s upper body strength by the number of push-ups they can perform. In this case, we decide to evaluate all children in all the elementary grades, from Kindergarten through fifth grade. We will assume that the average chronological age of these elementary school children is exactly eight years (CA=8-0 years).
After we test the third graders, we find that the average or mean score of our sample of 100 eight year old third graders is 6 push-ups. This means that the “average” third grade child (who is 8 years old) can do 6 push-ups. We can also compare an individual child’s score on arithmetic problems answered correctly with the average number answered correctly by children the same age.
How can we compare children from different groups? Let’s look at Larry who was a member of our original group of fifth graders. Although the average fifth grader performed 10 push-ups, Larry only completed 6 push-ups. His raw score of 6 converts to a percentile rank of nine (PR=9).
When we compare Larry’s performance to all elementary school students, we learn that Larry (a fifth grader) is functioning at the level of the average third grader—who is also eight years old—in the ability to do push-ups. Therefore, we see that Larry’s age equivalent score is 8 years (AE=8-0) and his grade equivalent score is at the third grade level (GE=3-0).
| Child’s Name | Raw Score | Percentile Rank |
|---|---|---|
| Oscar | 3 | 1 |
| Larry | 6 | 9 |
| Sam | 7 | 16 |
| Amy | 10 | 50 |
| Erik | 13 | 84 |
| Frank | 15 | 95 |
| Nancy | 17 | 99 |
Look at the table above and find Amy. At the time of testing, Amy was 10-0 years old and in the fifth grade. She scored at the mean for her peers, i.e., 10 push-ups. Her grade equivalent score was fifth grade (GE=5-0) and her age equivalent score was 10.0 years (AE=10-0). If we tested a 20 year old person and found that this person was able to do 10 push-ups, then the 20 year old has an age equivalent score of 10-0 and a grade equivalent score of 5.0, i.e., the same score as Amy.
Look again at the table of scores above and find Frank’s name. You see that Frank earned a raw score of 15 push-ups which converts to a percentile rank of 95 (PR=95). Frank’s score looks great—until we remember that Frank was “held back” three times. Although he is in the fifth grade, Frank is 13 years old!
With this new information, let’s take another look at Franks’ performance. The average score for 8th graders (who are 13 years old) is 15. Frank scored 15. Frank had a grade equivalent score of 8th grade (GE = 8.0) and an age equivalent score of 13 years (AE = 13-0). When we compare Frank with other children in his expected grade, we see that his achievement is in the average range. Frank is in the 95th percentile level when compared to fifth graders, not when compared to eighth graders.
Frank’s case brings up some additional questions. Frank (age 13) was included in our sample of 5 th graders who had an average age of 10. When compared to this group of children who were younger than him, Frank scored at the 95% percentile rank (PR) level. Question: If we compare Frank’s performance to that of children who are three years younger than him, will this comparison provide us with an accurate picture of his physical fitness? Answer: No.
In Frank’s case, statistics inform us of two facts. First, we see that Frank performs at a superior level when compared with other children in his grade. Second, we see that he performs at an average level when compared with children who are his age.
When you evaluate the significance of data from tests, you must know how the scores are being reported. Test scores can be reported using percentile ranks, age equivalents, grade equivalents, raw scores, scale scores, subtest scores, or standard scores.
Remember: Although Frank’s performance was superior for his grade, it was average for his age. If you did not know Frank’s age and grade, you would have been misled as to Frank’s actual achievement. But—if Frank was an 8 year old 3rd grader, his scores would be in the superior range, using both age equivalent and grade equivalent measures.
The number of push-ups each child completed was his or her raw score. Let’s assume that we want to obtain an overall fitness score. To obtain an overall or composite score, we will measure three skills (sit-ups, push-ups, a timed 50 yard dash) and obtain scores on each of these skills. In educational testing, the child’s overall score (in reading, math, etc.) is often a composite of several subtest scores.
Next, we will develop a weighting system that will convert each child’s raw score to a scale score. After we convert the raw scores to scale scores, we will be able to compare each of the three scores to each other (number of push-ups, number of sit-ups, seconds to complete the 50 yard dash). How do we convert raw scores into scale scores?
One way to convert scores is by developing a rank order system. In rank order scoring, the child who scores highest in an event (most push-ups, most sit-ups, fastest run) receives a scale score of 100; the lowest receives a score of 1. The other 98 children receive their respective “rank” as their scale score.
After each child’s raw scores are converted to scale scores, we can easily compare an individual child to the group and to all children who are the same age or in the same grade. We can also compare an individual child’s performance at different times, i.e. before and after completing the fitness course. Was the child able to do significantly more push-ups after taking the fitness course? Was the child reading better after receiving reading remediation?
Composite scores
You can see that after we develop a global composite score, the individual child’s raw scores on each of the three fitness subtests have less significance. This is exactly what happens with educational achievement and psychological tests. Most educational tests are composed of several subtests; the subtest scores are combined to develop composite scores. More about this shortly.
Let’s look at how composite scores can be used and some of the problems that arise when we rely on them.
John is a member of our original group of 100 fifth graders. He has good muscular strength (he scored at the 70% PR level in push-ups and at the 78% PR in sit-ups). But, John is very slow and uncoordinated. In the 50 yard dash, he finished 2nd from the last out of the 100 children (PR=2).
How will John’s composite fitness score be derived? In this example, we will average John’s percentile rank scores on the three events. John’s composite score is determined as follows: Add the percentile ranks of each event (70 + 78 + 2 = 150), then divide this score by the number of events (3). In John’s case, 150 / 3 = 50. (Note: actually it is improper to average the percentile rank scores, you must use the standard scores or scale / subtest scores.)
John’s composite score is 50. This composite percentile rank score of 50 places him squarely in the “average” range. Is John an “average” child? His individual scores demonstrated a significant amount of subtest scatter. When you analyze his three subtest scores, you see that he has specific strengths and a very severe deficiency. Despite his average composite score, John is not an average child! (Note: As noted above, the proper calculation is to use the standard scores. Thus the same analysis of John’s composite score by using standard scores is calculated to a standard score of 96.5 and percentile rank of 41—again, John appears to be an average child).
Let’s look at another example of composite scores to see how they can mislead us. Oscar was at the 1 percent level in push-ups. But when the other fitness subtests were given, Oscar was the fastest child in the class scoring at the 99% level. He was average in sit-ups, scoring at the 50% level. Oscar’s composite fitness score, using percentile ranking, is 50%. Is Oscar really an average child? Would he benefit from remediation to improve his upper body strength, as measured by push-ups? Oscar also has a great deal of subtest scatter, i.e., from extremely weak upper body strength to superior speed.
Subtest scatter
When subtest scores vary a great deal, this is called subtest scatter. If significant scatter exists, this suggests that the child has areas of strength and weakness that need to be explored.
How can you determine if significant subtest scatter is present? Most subtests have a mean score of 10. Most children will score + or - 3 points away from the mean of 10, i.e. most children will score between 7 and 13.
If the mean on a subtest is 10 (and most children score between 7 and 13), then scores between 9 and 11 will represent minimal subtest scatter. Lets assume that Child A is given a test that is composed of 10 subtests. The child’s scores on the 10 subtests are as follows: on 4 subtests, the child scores 10, on 3 subtests, the child scores 9, and on 3 subtests, the child scores 11. In this case, the overall composite score is 10 and the scatter is very minimal. This child scored in the average range in all 10 subtests.
In our next example, we will assume that Child B earns 4 subtest scores of 10, 3 scores of 4, and 3 scores of 16. The child did extremely well on 3 tests, very poorly on 3 tests, and average on 4 subtests. Again, the child’s composite score would be 10. Subtest scatter is the difference between the highest and lowest scores. In this case, subtest scatter would be 12 (16-4 = 12) Is this an “average” child? Because the child’s scores demonstrate very significant subtest scatter, we need to know more about these weak and strong areas.
In educational situations, it is essential that parents understand the nature of the weak areas, what skills need to be learned to strengthen those areas, and how the strong areas can be used to help remediate the child’s weak areas. The spread or variability between the subtest scores is called subtest scatter.
Apply your knowledge: Composite Scores & Subtest Scatter in the Wechsler Intelligence Scale for Children-IV (WISC-IV)
How do composite scores and subtest scatter relate to the information contained in your child’s evaluations? The results of educational tests given to children are often provided in composite scores.
The Wechsler Intelligence Scale for Children, Fourth Edition (WISC-IV) is the mostly commonly administered test of ability. The WISC-IV includes ten core subtests and five supplementary subtests. Each subtest measures a different ability.
Psychologists typically provide five scores: a Full Scale IQ (FSIQ) and four Index Scores - a Verbal Comprehension Index (VCI), a Perceptual Reasoning Index (PRI), a Working Memory Index (WMI), and a Processing Speed Index (PSI). Index Scores are composites of two or three subtest scores. The Full Scale IQ is a composite score that includes ten of the fifteen WISC-IV subtests.
IQ and Index Scores between 90 and 110 are considered within the “average range.” If there is a significant difference between Index scores or if there is significant scatter between subtests, the Full Scale IQ may not accurately represent the child’s level of functioning.
Katie is the 14 year old youngster whose situation was outlined earlier in this article. Let’s look at her scores on the subtests.
On the Wechsler Intelligence Scale for Children-IV, Katie achieved a Full Scale IQ of 101. If the only number you had was her Full Scale IQ score, you would probably assume that an IQ of 101 placed her squarely in the “average range” of intellectual functioning.
Is Katie an “average” child?
| Standard Score or Scaled Score | Standard Score or Scaled Score | ||
| WISC-IV Full Scale IQ | 101 | ||
| Verbal Comprehension Index | 124 | Perceptual Reasoning Index | 88 |
| Similarities | 16 | Block Design | 11 |
| Vocabulary | 14 | Picture Concepts | 7 |
| Comprehension | 12 | Matrix Reasoning | 6 |
| Information | (13) | Picture Completion | (8) |
| Word Reasoning | (12) | ||
| Working Memory Index | 110 | Processing Speed Index | 75 |
| Digit Span | 14 | Coding | 4 |
| Letter-Number Sequencing | 10 | Symbol Search | 7 |
| Arithmetic | (8) | Cancellation | (8) |
Remember: A Full Scale IQ score is a composite of four Index Scores (VCI, PRI, WMI, and PSI). When you look at Katie’s scores, you see that she has significant subtest scatter, from a high of 16 on the Similarities subtest (98th percentile) to a low score of 4 on Coding (2nd percentile). By using the Conversion Table below, you can convert the rest of her subtest scores.
There are also significant differences between Katie’s Index Scores. Her Verbal Comprehension Index Score (VCI) is 124 (95th percentile), while her Perceptual Reasoning Index Score (PRI) is 88 (21st percentile). When you subtract Katie’s Perceptual Reasoning Index Score (PRI) of 124 from her Verbal Comprehension Index Score (VCI) of 88, you find a 36 point difference between these Index Scores.
And, when you subtract her score on the Perceptual Reasoning Index - 21st percentile from her score on the Verbal Comprehension Index - 95th percentile. you see that a difference of 74 points in her percentile ranks (95-21=74) on the Index Scores.
If we rely on composite Index Scores or Full Scale IQ scores, we may easily be misled, with serious consequences. If we did not examine the subtest scores and Index Scores, we might view Katie as an “average” child - and we would be mistaken.
We will look at more of Katie’s test scores shortly.
Woodcock-Johnson Tests of Achievement (WJ-III ACH)
One of the most commonly administered individual educational achievement tests is the Woodcock Johnson III Tests of Achievement (WJ-III ACH). The Woodcock-Johnson III Tests of Achievement include two batteries, a standard battery and an extended battery. Subtests are organized into clusters.
Because the WJ-III subtests are short, many do not provide good qualitative information about what a child knows, can do, and where the child needs continued work. For example, the WJ-III does not measure the child’s ability to write a paragraph or an essay; it only examines the ability to formulate brief responses.
The WJ-III is scored by computer. The results obtained are organized into cluster scores. Cluster scores must be considered with caution when there is a significant difference between individual subtest scores. Relying on composite or ‘cluster’ scores can lead to faulty educational decision-making that have tragic consequences for children.
Tip: Parents must obtain all subtest scores on the tests that have been administered on their child and examine subtest scores and Index Scores.
When apparent progress means actual regression
One concern that many parents share is the belief that their child is not making adequate progress in a special education program. How can parents know if their perception is accurate? How can parents persuade school officials that the special education program being provided needs to be changed?
Earlier in this article, we discussed how statistics are used in medical treatment planning. We demonstrated how a medical problem is identified and the efficacy of treatment is measured by the use of objective tests. In our example, the patient had pre- and post- testing to determine if the intervention was working. Based on post test results, more medical decisions would be made—to continue, terminate, or change the treatment plan.
This practice of measuring change, called pre- and post- testing, is essential to educational planning. The child’s levels of performance are measured. An educational plan (IEP) is developed and implemented. The child is re-tested at set intervals to determine if the child is progressing, regressing, or maintaining the same position within the group (stagnating).
When we use pre- and post-testing, we can measure educational benefit (or lack of educational benefit). We can use scores from pre- and post- testing to create graphs to visually demonstrate the child’s progress or lack of progress in any academic area.
To see how this works, let’s visit our fifth grade fitness class. According to earlier testing in September, Erik completed 13 push-ups which placed him in the 84th percentile of all youngsters in his class. After a year of fitness training, fifth graders were re-tested. When Erik was re-tested, he completed 14 push-ups.
Question: Did Erik progress?
Answer: Yes and no.
The average performance of the fifth grade class improved by 2 push-ups, from an average raw score of 10 to 12. Erik’s raw score increased by 1 push-up, from 13 to 14. We see that while Erik’s age equivalent and grade equivalent scores increased slightly from earlier testing, his actual position in the group dropped from the 84th percentile to the 75th percentile. While Eric is still ahead of his peers, he regressed.
What about Sam? Sam’s performance also improved, from a raw score of 7 to 8. Although Sam’s age equivalent and grade equivalent scores increased slightly, he also regressed. He dropped from the 16th percentile to the 9th percentile. Sam continues to fall further behind the peer group.
Assume that we test Sam again, when he re-enters school in the fall. Now, we have three sets of test data (beginning 5th grade, end 5th grade, beginning 6th grade). Did Sam’s score change? If his percentile continues to fall, Sam continues to regress. We need to know how long will it take for Sam to recoup the skills he lost during the summer. Regression and recoupment are two of the issues considered when determining if the child needs Extended School Year (ESY) services during the summer.
Norm referenced and criterion referenced tests
Most standardized tests are norm referenced or criterion referenced.
When we evaluated our group of fifth graders, we compared each child’s performance to the norm group of fifth graders. Both Erik (raw score of 13, percentile rank of 84) and Sam (raw score of 7, percentile rank of 16) were compared to this norm group of fifth graders. To evaluate benefit, we looked at the norm group and the individual child’s position in that group when we administered the first and second tests. We computed each child’s change in position to determine progress or regression.
In our example, we also referenced the criteria of number of push-ups completed. A criterion reference analysis determines whether or not a child meets certain criteria (without reference to a norm group.) For example, at the beginning of the year, Sam completed 7 push-ups. If the criteria for success was 8 push-ups, Sam failed to reach that goal. Assume that Sam received a year of physical fitness remediation. After that year, Sam completed 8 push-ups. Does Sam met the criteria for success? The answer to this question depends on whether the criteria increased because Sam is a year older.
Another factor complicates this picture. We know that Sam’s’ peer group completed 10 push-ups at the beginning of the year and 12 push-ups at the end of the year. Definitions of success are affected by the passage of time. If we rely on criterion referenced measures, we can be misled as to whether the child is falling further behind the peer group. We need to know exactly what the criterion is and what this means when the child is compared to a norm group.
Standard deviation
Percentile ranks are computed by determining the mean score and the amount of variation of all scores around the mean score. Are the scores bunched around the number 10 in a tight uniform distribution? Are the scores evenly distributed? Do they peak and taper slowly, or do they bunch at the ends, without few or no scores in the middle? Is there a great variance, with the scores spread over a wide range, with two or more peaks? Is there a normal bell curve distribution of scores?
On our push-up test, most of the 5th graders earned scores around 10 push-ups, with an even distribution above and below 10 push-ups. If one-half of the children completed 5 push-ups, one-fourth completed 14 push-ups, and one-fourth completed 16 push-ups, the average or mean number of push-ups would still be 10! One-half of the children scored above 10 and one-half below 10.
In this case, the scores are not evenly distributed in a smooth curve above and below the mean score of 10. The variance is very large and would present a very unusual curve with a peak at 5, a drop to zero between 6 and 13, a jump at 14, a drop at 15, another jump at 16. This distribution of scores would not present a normal bell curve distribution.
Educational and psychological tests are designed to present normal bell curve distributions with predictable patterns of scores. We need to know the mean and standard deviation of the test. In most educational and psychological tests, the mean is 100 and the standard deviation is 15. (Mean = 100, SD = 15) On most subtests, the mean is 10; the standard deviation is 3. (Mean = 10, SD = 3) Average scores do not deviate far from the mean. When a score falls significantly above or below the mean, it is referred to as being a distance from the mean, e.g., 1 or 2 standard deviations from the mean.
In all tests, the mean is 0 (zero) standard deviations from the mean. The next marker on the bell curve is +1 and -1 standard deviations from the mean, followed by 2 standard deviations from the mean. To interpret your child’s test scores, you need to know the mean and standard deviation.
Using our original push-up example, the mean was 10 push-ups. The standard deviation (SD) was 3 push-ups. This push-up example is identical to the subtest scores in almost all standardized educational and psychological testing.
One standard deviation above the mean is 10 plus 3, i.e. 10 + 3 = 13. One standard deviation below the mean is 10 minus 3; i.e. 10 - 3 = 7. One standard deviation above the mean always falls at the 84 percentile (PR = 84); one standard deviation below the mean is always at the 16 percentile (PR = 16). Two SD’s above the mean is always at the 98 percentile (PR = 98); and two SD’s below the mean are always at the 2nd percentile(PR = 2).
Click to see Bell curve graph 4(opens in a new window)
When we look at actual test scores, we see that the child scored “one standard deviation below the mean” on a particular test or subtest. If the score is one standard deviation below the mean, the child’s percentile rank is 16.
Remember: Most subtests have a mean of 10 and standard deviation of 3. If a child scores 7 on a subtest, this score is at the 16th percentile. A subtest score of 13 is at the 84th percentile.
Standard scores
One of the most difficult concepts for most people to grasp is standard scores. Since educational test scores are usually provided in standard scores, parents must know what they mean.
At an IEP meeting, a parent is told that the child earned a standard score of 85 in one area, a standard score of 70 in another area. Most parents are relieved to hear this news. Why? Most parents believe these numbers are similar to grades, with 100 as the highest score and 0 as the lowest. Standard scores are NOT like grades.
With standard scores, the average score or mean is 100. The standard deviation is 15. The average child earns a standard score of 100. If a child scores 1 standard deviation above the mean, the standard score is 100 plus 15; i.e. 100 + 15 = 115. If the child scores 1 standard deviation below the mean, this is 100 minus 15, i.e. 100 - 15 = 85.
A standard score of 115 is 1 standard deviation above the mean so it is always at the 84th percentile. A standard score of 85 is 1 standard deviation below the mean so it is always at the 16th percentile. A standard score of 130 (+2 SD) is always at the 98th percentile. A standard score of 70 (-2 SD) is always at the 2nd percentile.
Remember Katie? Earlier, we learned that on the Wechsler Intelligence Scale, Katie earned a Full Scale IQ of 101. Later, we realized that this score was misleading because Katie’s Verbal Comprehension Index Score (VCI) was 124, while her Perceptual Reasoning Index Score (PRI) was 86. The psychologist found that Katie scored 2 standard deviations above the mean on the Similarities subtest of the Wechsler Intelligence Scale for Children, 4th Edition (WISC-IV). What does this mean?
You learned that a score of 2 standard deviations above the mean places the child at the 98th percentile in the area being measured. Since the Similarities subtest of the WISC-IV measures verbal reasoning ability, Katie’s verbal reasoning power is at the 98 percentile.
The psychologist also found that Katie had a standard score of 68, 2.5 standard deviations below the mean, on the spontaneous writing sample of the Test of Written Language (TOWL-3). Two SD’s below the mean is at the 2nd percentile. With your new knowledge, you know that Katie’s ability to produce spontaneous writing samples was actually below the 1st percentile.
When we first introduced Katie, we posed two questions:
- Do these two test scores help to explain the academic problems Katie is having?
- Do her test scores tell us anything about her moodiness and her intense dislike of school?
Katie’s verbal reasoning ability places her at the 98th percentile of youngsters her age. However, her ability to convey her thoughts in writing is below the 1st percentile. Katie is very bright but she is unable to convey her knowledge to her teachers on written assignments and tests. Would you expect her to feel frustrated and stupid? Do you question why, after years of frustration, Katie is angry, depressed and now wants to quit school?
Wrightslaw quick rules of tests
All educational and psychological tests that report scores using percentile ranks or standard scores are based on the bell curve. To interpret tests results, you must know the mean and the standard deviation. Most standardized tests use a mean of 100 and a standard deviation of 15.
- When educational and psychological tests use standard scores (SS) with a mean of 100 and a standard deviation of 15, a standard score of 100 is at the 50% percentile (PR). A standard score of 85 is at the 16th percentile (PR=16) A standard score of 115 is at the 84th percentile (PR=84).
- When educational and psychological tests use subtest scores with a mean of 10 and standard deviation of 3, a subtest score of 10 is at the 50th percentile (PR=50).
- A subtest score of 7 is at the 16th percentile; a subtest score of 13 is at the 84th percentile (PR=84).
- A standard score of 100 is at the 50th percentile level. One-half of children will fall above and one- half will fall below the mean at the 50th percentile which is represented as a standard score of 100.
- Two-thirds of children will score between + 1 and - 1 standard deviations from the mean.
- Two-thirds of children will score between the 16% and 84% percentile ranks. (84 minus 16 = 68)
- Half of 68 percent is 34 percent. When you subtract 34 percent from the mean of 50 percent, you have 16 percent. When you add 34 percent to 50 percent, you have 84 percent.
- A standard deviation of -1 is at the 16th percentile. A standard deviation of 0 is at the 50th percentile. A standard deviation is +1 is at the 84th percentile.
- A standard score of 85 is at the 16th percentile. A standard score of 100 is at the 50th percentile. A standard score of 115 is at the 84th percentile.
- A standard deviation of -2 is at the 2nd percentile. A standard deviation of +2 is at the 98th percentile.
- A standard score of 70 is at the 2nd percentile. A standard score of 130 is at the 98th percentile.
- A standard score of 90 is at the 25th percentile. A standard score of 110 is at the 75th percentile.
- One-half (50 percent) of children will score between the 75th and 25th percentile. (75-25 = 50)
- One half (50 percent) of children will have standard scores between 90 to 110.
- A percentile rank score between 25% and 75% is the same as a standard score of between 90 to 110, which is within the “average range.”
The results of most educational tests are reported as standard scores. Parents must learn how to convert standard scores into percentile ranks. By using the conversion table(opens in a new window) and the bell curve, you can convert any standard score into a percentile rank. The earlier push-up example used standard scores. Click here to view the table.(opens in a new window)
Understanding test data
Means and standard deviations of other tests
With some tests, scores are reported differently. For example, test scores may be reported as “z scores.” Z scores are have a mean of 0 (zero) and and standard deviation of 1 (Mean = 0, SD = 1)
If you know that a child earned a z score of -1, you know that the child scored one standard deviation below the mean. One standard deviation below the mean is at the 16th percentile. If you convert this score into the standard score format, with a mean of 100 and a standard deviation of 15, a z score of -1 is the same as a standard score of 85.
Other tests report results as T Scores. T scores have a mean of 50 and a standard deviation of 10 (Mean =50; SD=10). A T score of 60 is the same as a z score of +1. A child who has a T score of 60 or a Z score of +1 scored at the 84th percentile rank. A T score of 70 is the same as a z score of +2, a standard score of 130, and a percentile rank of 98.
A few tests report results in Stanines. In Stanine tests, the mean is five and the standard deviation is 2 (Mean = 5; SD=2).
Applying your knowledge to subtests
Since tests are always in a state of change with new editions being published, we will not attempt to review and describe tests in this article. Please check the links at the end of this article for test information.
Earlier, you learned that Index Scores are actually composites or averages of two or three different subtests. Each subtest measures different abilities. Let’s take look at Katie’s subtest scores to see what we can learn from them.
| Standard Score or Scaled Score | Standard Score or Scaled Score | ||
| WISC-IV Full Scale IQ | 101 | ||
| Verbal Comprehension Index | 124 | Perceptual Reasoning Index | 88 |
| Similarities | 16 | Block Design | 11 |
| Vocabulary | 14 | Picture Concepts | 7 |
| Comprehension | 12 | Matrix Reasoning | 6 |
| Information | (13) | Picture Completion | (8) |
| Word Reasoning | (12) | ||
| Working Memory Index | 110 | Processing Speed Index | 75 |
| Digit Span | 14 | Coding | 4 |
| Letter-Number Sequencing | 10 | Symbol Search | 7 |
| Arithmetic | (8) | Cancellation | (8) |
Wrightslaw Note: Scores in Brackets ( ) are supplementary subtests. They are not used to calculate the Full Scale IQ or Index Scores.
When we presented Katie’s test results, you learned that variation among subtest scores (subtest scatter) is a valuable source of information. Look at Katie’s WISC-IV Index and subtest scores in the table above. You can see that she has significant subtest scatter, from a high score of 16 on Similarities (98th percentile) to a low score of 4 on Coding (2nd percentile).
Subtests of the WISC-IV range from a low score of 1 to a high score of 19. WISC-IV subtest scores have a mean of 10 and a standard deviation of 3. A subtest score of 7 is one standard deviation below the mean (-1 SD). By using the Conversion Table, you can convert the subtest score of 7 to a percentile rank of 16 (PR = 16). You can also convert the subtest score of 7 to a standard score of 85.
When you look at Katie’s subtest scores, you see that she has significant subtest scatter, from a high score of 16 on the Similarities subtest (98th percentile) to a low score of 4 on the Coding subtest (2nd percentile). You know that subtest scatter is the difference between the highest and lowest subtest scores. Subtract the lowest score of 4 (Coding) from her highest score of 16 (Similarities). Katie’s subtest scatter is 12 (16 - 4 = 12). The WISC-IV manual tells us that scatter this great is unusual.
You need to understand what subtests measure. When we first discussed Katie’s test scores, you learned that Similarities subtest is highly correlated with abstract reasoning.
The Coding subtest measures visual-perceptual mechanics. Assessment experts Jerome Sattler and Ron Dumont (information is at the end of this article) describe the Coding subtest as “an information processing task that involves the discrimination and memory of visual pattern symbols.”
If you find that a child has a visual, hearing, attentional, or motor problem that may interfere with his or her ability to take one or more of the subtests, do not use these subtests in computing Index scores or a Full Scale IQ score. A left handed child may be penalized on the Coding subtest because the child will “have to lift his hand repeatedly during the task to view” the test items.
Katie’s scores are evidence that she could excel in discussions of complex literature in an honors English class because of her reasoning abilities, but she is unable to write what she knows. Since Katie cannot write what she knew, she was placed in slow-paced remedial classes. Because her abilities were untapped, Katie concluded that she was stupid and wanted to quit school.
When you look at Katie’s subtest scores, you see that several scores are in parentheses. On the WISC-IV, Information, Word Reasoning, Arithmetic, Picture Completion, and Cancellation are not included in the Full Scale IQ or the Index Scores. These subtests are used to provide additional data about how a child learns.
Subtests of the Wechsler Intelligence Scale for Children-IV (WISC-IV)
The WISC-IV Technical and Interpretive Manual describes the WISC-IV subtests as follows:
| Indexes & Subtests | Ability Measured |
| Verbal Comprehension Index | |
| Similarities | Abstract reasoning, verbal categories and concepts |
| Vocabulary | Language development, word knowledge, verbal fluency |
| Comprehension | Social and practical judgment, common sense |
| Information (supplementary) | Factual knowledge, long-term memory, recall |
| Word Reasoning (supplementary) | Verbal comprehension, general reasoning ability |
| Working Memory Index | |
| Digit Span | Short-term auditory memory, mental manipulation |
| Letter-Number Sequencing | Sequencing, mental manipulation, attention |
| Arithmetic (supplementary) | Attention and concentration, numerical reasoning |
| Perceptual Reasoning Index | |
| Block Design | Spatial analysis, abstract visual problem solving |
| Picture Concepts | Abstract, categorical reasoning |
| Matrix Reasoning | Pattern recognition, classification, analogical reasoning |
| Picture Completion (supplementary) | Alertness to detail, visual discrimination |
| Processing Speed Index | |
| Coding | Visual-motor coordination, speed, concentration |
| Symbol Search | Visual-motor quickness, concentration, persistence |
| Cancellation (supplementary) | Processing speed, visual selective attention, vigilance |
Psycho-educational evaluations by evaluators in the private sector
We find that public school evaluators are often limited in the tests that are available for their use. Heavy workloads may prevent them from completing a comprehensive evaluation of a child. As a result, we do not rely on testing by public school employees. Instead, we have the child evaluated by a child psychologist, school psychologist, speech language pathologist, and/or educational diagnostician in the private sector.
A word about Individualized Education Programs (IEPs)
When you use this article and Wrightslaw: Special Education Law, Second Edition, you will be able to write IEP’s that include measurable goals.
After you master the information in this article, you will be able to convert test scores into easily understood numbers. You will be able to measure and monitor your child’s educational progress. The feelings of helplessness and confusion you have experienced at school meetings will dissipate. You will be knowledgeable about your child’s test scores and the significance of the data.
As Susan Bruce learned, “the numbers don’t lie.” To learn how Susan used information from this article and Wrightslaw: Special Education Law to get quality special education programs for her children, read Success Story: From Victim to a Mighty Force.
The parents' "to-do list"
- After you complete this article, make a list of all the times when your child has been tested. Arrange your list in chronological order. Include the names, dates, and scores of each test that has been administered to your child more than once.
- Begin your list with the test or tests that have been administered most frequently. In many cases, that will be the Wechsler Intelligence Test and the Woodcock-Johnson and/or Kaufmann Educational Achievement Tests.
- Write down all of the scores from the first administration of a test battery. Convert these scores to percentile ranks. Complete the same process with the most recent testing of the same battery. Compare the results. You should be able to determine whether your child is being remediated (catching up), staying in the same position, or falling further behind the peer group.
- Dig for the standard scores or percentile rank scores in your child’s file. You may find that some scores are only reported in “ranges” (i.e., high- average, low-average) or in grade equivalent or age equivalent scores. If the standard scores are not available, you should ask for them. When you request the data in standard score format, the school staff may be surprised but they should be able to comply with your request.
- Take the most glaring deficiencies where your child has shown minimal progress or even regression and chart out the test results. If you do not have a computer, use graph paper. Software programs like Excel and PowerPoint allow for dramatic visual presentations of test data. If this is too difficult or confusing, consult with an expert.
Gather your material—your bell curve chart and standard score / percentile rank chart, your list of test scores, and your child’s evaluations, and consult with a private sector psychologist or educational diagnostician who can explain the significance of the scores using percentile ranks. - Ask the professional to use the bell curve chart that includes standard scores, standard deviations and percentile ranks. Be sure that you have a photocopy of the bell curve so you can take it home to study. If the professional is willing, it may be helpful to tape record this portion of the session so that you can go back over it at home with the test scores in front of you.
- Contact your state’s Department of Education and request all publications about special education and IEPs, along with your state regulations.
- Download our companion article, “Your Child’s IEP: Practical and Legal Guidance for Parents and Advocates.”
Resources about testing
- Testlink(opens in a new window)
For information about thousands of tests, go to Testlink from the Educational Testing Service (ETS) - Reading Tests: What They Measure, and Don’t Measure(opens in a new window)
By Dr. Melissa Farrall — “There are two important realities in testing. Tests do not always measure what they appear to measure, and not all tests measure reading, writing, and math skills comprehensively.”
Bell curve charts & percentile rank / standard scores conversion charts
Download and print bell curve charts and a list of standard scores, scale / subtest scores, standard deviation and percentile ranks:
Print several copies of both. You will be surprised at how often you refer to them. Make copies for your friends.
Don’t forget to download Your Child’s IEP: Practical and Legal Guidance for Parents and Advocates(opens in a new window).
Books
- Wrightslaw: From Emotions to Advocacy, 2nd Edition(opens in a new window)
Chapters 10 and 11 teach you about tests and measurements and how to measure progress objectively. From Emotions to Advocacy includes bell curves, charts, graphs, and other visual aides to help you master this subject.
Chapter 12 about SMART IEPs teaches you how to draft IEPs that are Specific, Measurable, use Action words, are Realistic, and Time Specific. - Assessment of Children: Cognitive Applications (4th edition)(opens in a new window)
By Jerome M. Sattler. Excellent book about assessments and tests for the professional, attorney, and the curious parent. Dr. Sattler’s book includes a Bell Curve with percentile ranks for the Wechsler IQ tests, subtest scores, and most other tests that are used with children who receive special education services. - Assessment of Children: WISC-IV and WPPSI-III Supplement(opens in a new window)
By Jerome M. Sattler. You can order Dr. Sattler’s books from his publishing site, or from the Advocate’s Bookstore at Wrightslaw.
Wrightslaw Note: This comprehensive article was updated and revised in December 2007. For more information about the bell curve and how to use tests and measurements to measure and monitor a child’s progress, please read Chapters 10 and 11 in Wrightslaw: From Emotions to Advocacy, 2nd Edition.
Wrightslaw: From Emotions to Advocacy(opens in a new window), 2nd Edition includes a new section about the WISC-IV, changes from the WISC-III, and additional information about tests commonly administered to children, including intelligence / cognitive tests, educational achievement tests, speech language tests, etc. Click here(opens in a new window) to learn more about Wrightslaw: From Emotions to Advocacy, 2nd Edition.
Used with permission from Peter W. D. Wright, Esq. and Pamela Darr Wright, M.A., M.S.W. Licensed Clinical Social Worker. Wright, P.W.D., and Wright, P.D. (2007). Tests and Measurements for the Parent, Teacher, Advocate, and Attorney. Retrieved online, April 11, 2008, from http://www.wrightslaw.com/advoc/articles/tests_measurements.html.